Guest Post by Huan Lee, Lambient Technologies
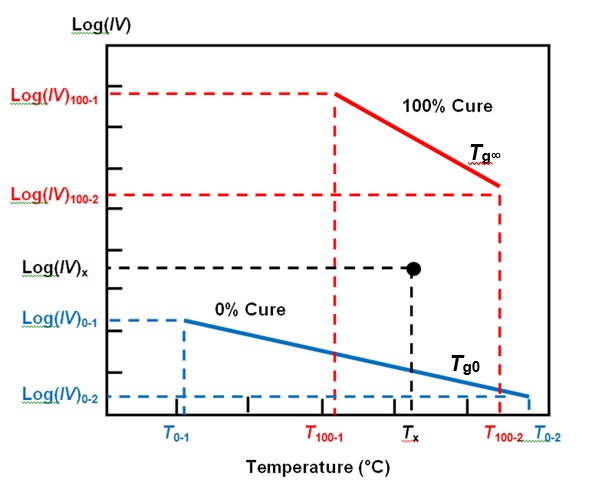
In my last post we saw how Cure Index accounts for the effect of temperature on ion viscosity and yields a value related to cure state. For a given material, any measurement of ion viscosity and the associated temperature exists as a point between two baselines: one representing the temperature dependence of ion viscosity at 0% cure and the other at 100% cure, as shown in Figure 1.
Figure 1. Cure Index baselines
Cure Index, given by Equation 1, is the vertical distance between this point and the 0% baseline, expressed as a proportion of the vertical distance between the two baselines.
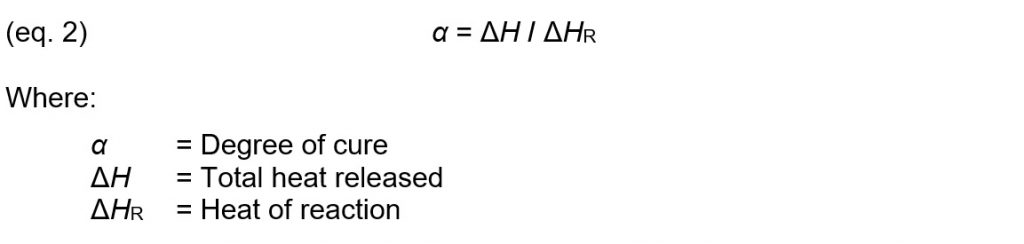
How does Cure Index relate to degree of cure? As a thermoset reacts and polymerizes, each bond releases a fixed amount of heat. Degree of cure is defined as:
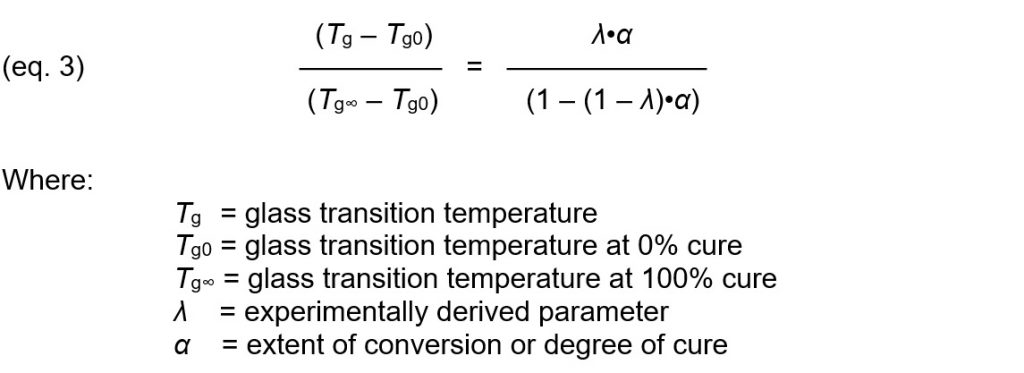
Degree of cure therefore can indicate physical state because it measures the total amount of bond formation or crosslink density. Furthermore, Ueberreiter and Kanig [Ref. 1] reported crosslink density is directly proportional to the change in glass transition temperature. This relationship between degree of cure and glass transition temperature is often expressed by the DiBenedetto equation [Ref. 2]:
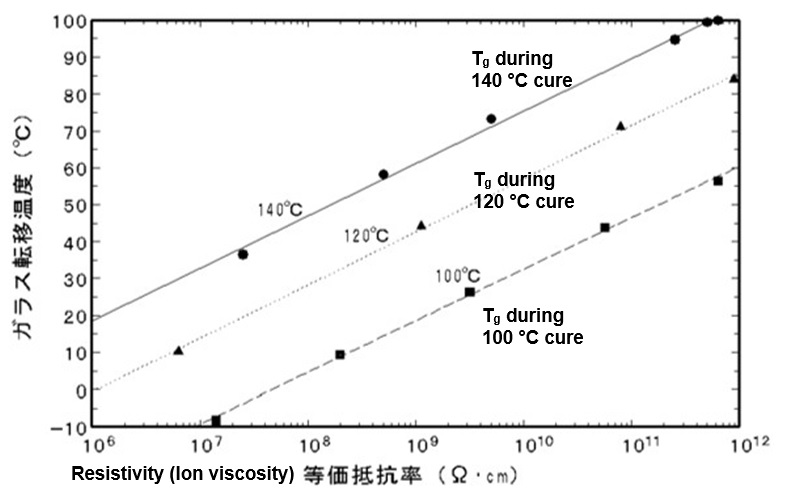
Because ion viscosity increases with crosslink density, dielectric measurements should correlate with glass transition temperature. Several published studies, such as the epoxy data of Figure 2, show a linear relationship between log(IV) and Tg.
Figure 2. Glass transition temperature vs. resistivity (ion viscosity) for an epoxy cured at 100 °C, 120 °C and 140 °C [Ref. 3]
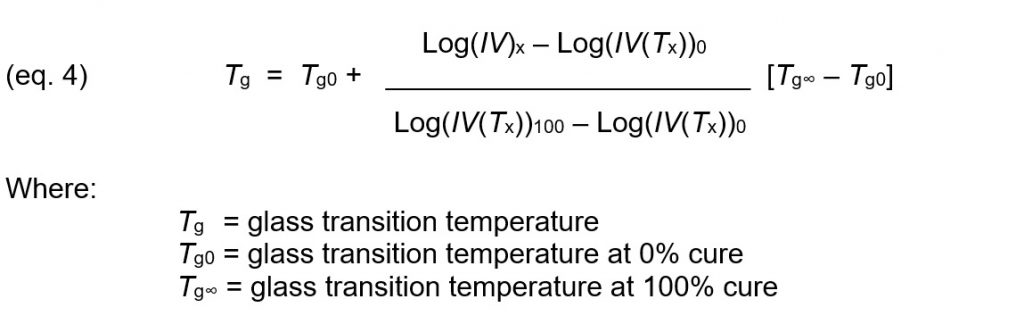
Assuming the linear relationship between Tg and log(IV) shown in Figure 1, the glass transition temperature as a function of Tx and Log(IV)x, can be calculated by linear interpolation:
Equations 1 and 4 may be combined and rearranged to yield Cure Index, which is a reproducible indicator of cure state that does not rely on glass transition temperature but correlates with glass transition temperature.
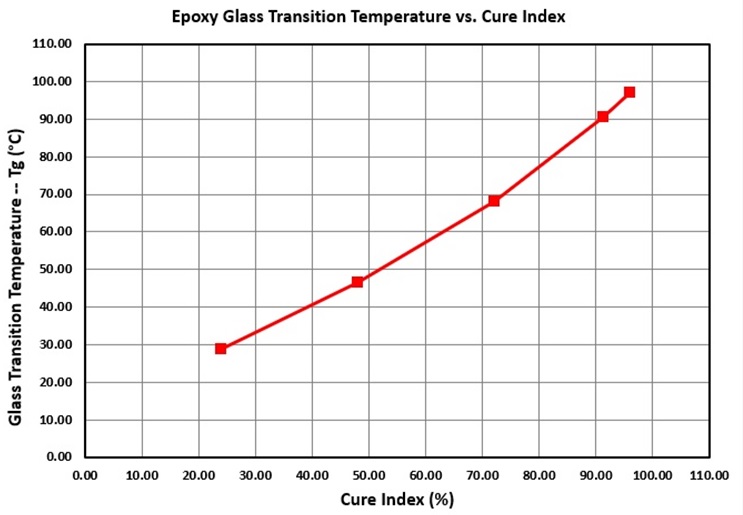
Figure 3 shows how the glass transition temperature of an epoxy resin changes with Cure Index during cure. As with the epoxy of Figure 2, a linear or near-linear relationship exists between Tg and Cure Index.
Figure 3. Glass transition temperature vs. Cure Index during an epoxy cure
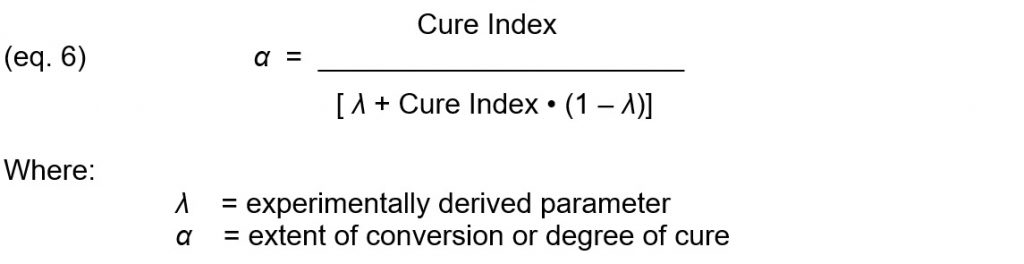
The DiBenedetto equation (eq. 3) can combined with the definition of Cure Index (eq. 5) and rearranged to express degree of cure in terms of Cure Index:
Equation 6 shows degree of cure can be derived from Cure Index and therefore from ion viscosity and temperature. If the experimentally derived parameter λ = 1, then equation 6 becomes:
Unlike differential scanning calorimetry (DSC) or dynamic mechanic analysis (DMA), which can only be used in the laboratory, dielectric cure monitoring (DEA) works in molds, presses or manufacturing operations. With Cure Index dielectric cure monitoring has the unique ability to make measurements that correlate with glass transition temperature and degree of cure in-process and in real time.
References
- Ueberreiter, K.; Kanig, G., Journal of Chemical Physics, 18, 399 (1950).
- Pascault, J.P. and Williams, R.J.J., “Glass transition Temperature vs. Conversion Relationships for Thermosetting Polymers,” J. of Polymer Science, Part B:Polymer Physics, 28, 85-95 (1990).
- Makishima, S.; Nakano, T.; Koyama, M.; Inoue, Y., “Dielectric Property of Curing Epoxy Resin and Application for Casting Process,” IEEE Electrical Electronics Insulation Conference, Sept. 18-21, 1995
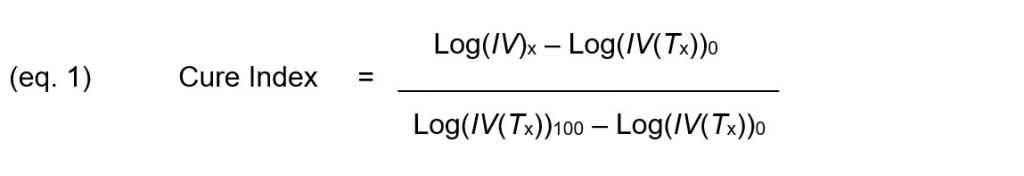
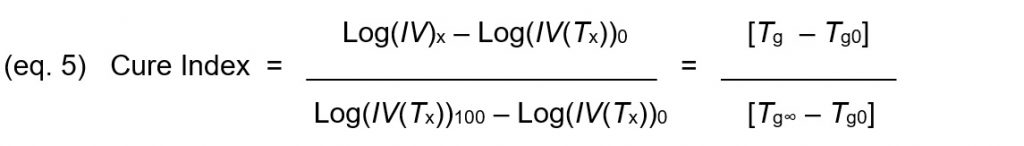

Leave a Reply