Guest Post by Dr. R. Bruce Prime
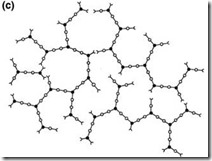 In the last post we introduced the concept of gelation. Since gelation is the practical endpoint during thermoset processing, it is important to understand how the chemistry and stoichiometric ratios impact the conversion at the gel point.
In the last post we introduced the concept of gelation. Since gelation is the practical endpoint during thermoset processing, it is important to understand how the chemistry and stoichiometric ratios impact the conversion at the gel point.
First to review, gelation
- is the point during cure where a viscous liquid becomes a cross-linked gel or rubber
- is isoconversional, i.e. agel is constant for a given thermoset, independent of temperature
- does not affect the rate of cure, and for this reason is not detected by DSC
- is determined precisely as the point where tan d becomes frequency-independent in a dynamic rheology measurement
- is approximated by the G’ = G” crossover in a dynamic rheology measurement, commonly at 1 Hz, or by achievement of a very high viscosity
Gelation is dependent on the functionality, reactivity and stoichiometry of the reactants. The gel point may be calculated if the chemistry is known (1,2). Gelation in condensation systems like epoxy-amine typically occurs between 50 and 80% conversion (a = 0.5 – 0.8). For high functionality and free-radical initiated systems, gelation will typically occur at lower conversions.
The conversion at the gel point can be calculated using the following equation:
where:
Take the simple diepoxy-diamine system with equal stoichiometry, where in the above equation:
a1 = conversion of amine
a2 = conversion of epoxide
f1 = functionality of amine = 4
f2 = functionality of epoxy = 2
equal stoichiometry then a1,gel = a2,gel = agel
leading to:
![]()
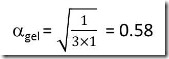 , i.e. the stoichiometric diepoxy-diamine gels at 58% conversion of both epoxy and amine groups.
, i.e. the stoichiometric diepoxy-diamine gels at 58% conversion of both epoxy and amine groups.
It is more likely that an epoxy-amine system will have unequal stoichiometry, which is accounted for by the term B, the number or equivalents of amine hydrogens to the number or equivalents of epoxide groups. For equal stoichiometry B = 1, for a system with excess amine B > 1 and for excess epoxy B < 1.
Consider a system with 30% excess amine, i.e. B = 1.3. Assuming that the minor component will completely react and the excess of the major component will remain unreacted leads to a2 = Ba1, or in this example a2 = 1.3a1. Substituting in the equation above gives:
In a similar manner:
Thus for a diepoxy-diamine system with 30% excess amine gelation will occur when 66% of the epoxide and 51% of the amine have reacted.
Finally, consider a thermoset consisting of 25% excess tetrafunctional epoxide reacting with a diamine. Here B = 1/1.25 = 0.8,
Thus for a diepoxy-diamine system with 30% excess amine gelation will occur when 66% of the epoxide and 51% of the amine have reacted.
Finally, consider a thermoset consisting of 25% excess tetrafunctional epoxide reacting with a diamine. Here B = 1/1.25 = 0.8, a2 = 0.8a1, and both f1 and f2 = 4. Again substituting in the equation above gives:
A tetraepoxy-diamine system with 25% excess epoxy will gel at much lower conversion when 30% of the epoxide and 37% of the amine have reacted.
While gelation cannot be measured by DSC directly, the time to gel can be established as the time to reach agel provided this quantity can be calculated.
In a large number of thermosets the composition will not be known, precluding calculation of agel. In these cases common means to measure gelation are dynamic rheology and dynamic mechanical analysis (DMA), both of which are subjects of future posts.
References
1. P. J. Flory, J. Am. Chem. Soc. 63, 3083 (1941).
2. P. J. Flory, Principles of Polymer Chemistry, Cornell Univ. Press, Ithaca, NY (1953).
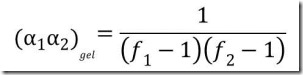
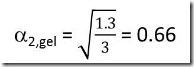
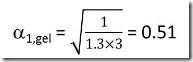

Hi Jeff,
Great overview!
I don’t suppose you happen to have a spreadsheet that you could share to conduct these calculations for polyester polyols?
Many thanks and best,
Rick